We will determine ![]() for any monotonically decreasing function
for any monotonically decreasing function ![]() .
Since g is monotontically decreasing,
.
Since g is monotontically decreasing, ![]() .
We assume that
.
We assume that ![]() ,
and that
,
and that ![]() , where
, where ![]() ;
;
![]() , so that
, so that ![]() .
Take
.
Take ![]() ; a simple proof
by contradiction, which follows, shows that
; a simple proof
by contradiction, which follows, shows that
![]() is an upper bound for g:
is an upper bound for g:
![]()
Assume that there is a point ![]() such that
such that ![]() .
Let
.
Let ![]() , so
, so ![]() .
Furthermore,
.
Furthermore, ![]() and
and ![]() imply that
imply that ![]() .
.
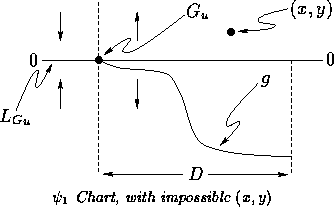
| Jeff Tupper | March 1996 |